A new rheology that explicitly accounts for the sub-continuum anisotropy of the sea ice cover is implemented into the Los Alamos sea ice model (CICE). This is in contrast to all models of sea ice included in GCMs (Global Circulation Models) that use an isotropic rheology. The model contains one new prognostic variable, the local structure tensor, that quantifies the degree of anisotropy of the sea ice, and two parameters that set the time scale of the evolution of this tensor. The anisotropic rheology provides a sub-continuum description of the mechanical behaviour of sea ice and accounts for a continuum scale stress with large shear to compression ratio and tensile stress component. Results over the Arctic of a stand-alone version of the model are presented and anisotropic model sensitivity runs are compared with a reference elasto-visco-plastic simulation. Under realistic forcing sea ice quickly becomes highly anisotropic over large length scales, as is observed from satellite imagery. The influence of the new rheology on the state and dynamics of the sea ice cover is discussed. Our reference anisotropic run reveals that the new rheology leads to a substantial change of the spatial distribution of ice thickness and ice drift relative to the reference standard visco-plastic isotropic run, with ice thickness regionally increased by more than one meter, and ice speed reduced by up to .
[id="" class="" style=""]
The rapid decrease of the Arctic sea ice extent and volume over recent years raises questions as to the importance of this decline both in the global climatic system and at the regional scale. An important contribution to our understanding of this changing region of the world lies in the correct description of the rheological and mechanical properties of the Arctic ice cover. While the determination of suitable constitutive relations to describe sea ice rheology remains an outstanding problem that limits the success of sea ice models [Pritchard, Feltham2008]\citep{Pritchard2001,Feltham2008}, the climate modeling community has converged, as evidenced by the latest International Panel on Climate Change Fourth Assessment Report (IPCC AR4), to the almost exclusive use of a rheology of the viscous-plastic type (VP) \citep{Hibler1979} based upon the earlier AIDJEX model \citep{Coon1974}. A commonly used example of a VP rheology is the elastic-visco-plastic (EVP) rheology that is used in the Los Alamos CICE sea ice model \citep{Hunke2008}. \citet{Kreyscher2000} showed that this type of rheology performs better in reproducing the spatial pattern and average thickness of sea ice as well as the regional ice drift and Fram strait outflow, than other, more crude, descriptions of the rheology where the ice pack is in free drift, behaves as a compressible viscous Newtonian fluid, or has no shear strength.
Nevertheless important differences between the results from models using a VP rheology and observations remain. Comparison with ice thickness obtained from submarine cruises have shown simulated ice that is too thick in the Beaufort sea and too thin near the North Pole \citep{Miller2005, Kreyscher2000}. Detailed analysis of ice motion using the RADARSAT Geophysical Processor System (RGPS) \citep{Kwok2008} shows that models underestimate ice drift off the coast of Alaska and Siberia, poorly reproduce time series of shear, vorticity and particularly divergence at the regional scales, and consistently underestimate deformation-related ice volume production. \citet{Rampal2011} argues that this deficiency of current IPCC climate models to accurately capture the coupling between the ice state and dynamics could partly explain the models underestimation of the recent sea ice area, thickness, and velocity trends.
The discrepancies between models and observations suggest that one needs to question the underlying assumptions used in the development of the VP models (e.g. \citet{Coon2007}). First, with new computer capabilities sea ice models can be routinely run at length scales of 10 km or less, but since sea ice is composed of polygonal floes (Figure \ref{Fig1}) of sizes ranging from tens of meters to tens of kilometres, this raises the question of the validity of a continuum description of sea ice at these scales. The issue of the validity of the continuum assumption, closely tied to the scale dependence of the rheology, is discussed in \citet{Feltham2008} Supplemental Appendix C. Discrete element approaches, which resolve individual floes, have been used to study sea ice dynamics but remain computationally unsuitable for climate simulation, e.g. \citep{Hopkins2004, Wilchinsky2011}.
Second, in contrast with in situ data obtained during the SHEBA and SIMI experiments \citep{Coon1998, Weiss2007a}, early versions of the VP rheology did not account for the capacity of sea ice to withstand tension. \citet{Hibler1979} showed that doubling the shear strength in an isotropic model reduced the flow over the Arctic basin, causing a reduction of outflow and ice drift and an increase of the average ice thickness by about $5\%$. More recently \citet{Zhang2005}, \citet{Miller2005} and \citet{Wilchinsky2006c} showed that by modifying the shape of the yield curve, for example by increasing the shear strength or by incorporating biaxial tensile stress, a better agreement with observed ice thickness distribution could be achieved.
Third, most currently used models of sea ice do not treat explicitly the sea ice elasticity. Yet \citet{Wilchinsky2010, Wilchinsky2011} and \citet{Girard2011} have recently shown that accounting for elasticity, in particular through the long range interactions that it allows in the ice cover, enables models to more accurately reproduce the degree of localisation of the sea ice deformation.
%Finally, and more crucially for this article, the hypothesis of isotropy is inaccurate below 100 km, the resolution at which most models are run today
Finally, and more crucially for this article, the hypothesis of isotropy was shown to be inaccurate at the resolution at which most models are run today. Figure \ref{Fig1}, a $50\times50$ km$^{2}$ optical image from the LANDSAT-7 satellite \citep{Weiss2009}, shows a typical sea ice area composed of various fractures and faults. In this area of sea ice, which coincides with the typical grid size used in sea ice models, a network of intersecting leads imparts to the sea ice cover a preferred orientation with floes of roughly diamond geometry, aligned vertically along the figure. The effect of anisotropy has been treated either explicitly through consideration of particular leads \citep{Coon1998,Pritchard1998,Hibler2000, Hibler2001,Schreyer2006,Wilchinsky2011} or implicitly through continuum representation of anisotropy using heuristic arguments \citep{Wilchinsky2004b, Wilchinsky2006b}. Additionally, \citet{Wilchinsky2006a}, motivated by satellite imagery, treated the ice cover as comprising diamond-shaped ice blocks formed from intersecting slip lines, to develop an anisotropic sea ice model avoiding detailed modeling of fracture processes. In this paper we do not resort to the assumption of isotropy and implement into the Los Alamos CICE sea ice model a new rheology \citep{Wilchinsky2006a} that explicitly accounts for the sub-grid scale anisotropy of the sea ice cover. We use this model to perform the first continuum Arctic basin scale simulation of sea ice that explicitly accounts for the sub-continuum anisotropy of the sea ice cover.
The paper is structured as follows: In section \ref{anisotropic_model} we introduce the anisotropic model and discuss issues relevant to its implementation in the Los Alamos CICE sea ice model. In section \ref{arctic_simulations} we apply our model to the Arctic Ocean using realistic forcing. We compare our anisotropic sea ice model simulations to those obtained with the standard, isotropic sea ice model and discuss how the introduction of anisotropy changes the simulated sea ice mechanical behaviour.
We then proceed, in section \ref{sensitivity}, to a sensitivity study demonstrating the impact of the new anisotropic model parameters. Finally, in section~\ref{conclusions} we summarize our main results and discuss some implications of our work.
From a more mathematical point of view, inputs to the neurons are described as ionic currents flowing through the cell membrane when neurotransmitters are released. Their sum is seen as a physical time-dependent current and the membrane is described as an
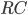 circuit, charged by (see Figure taken from [BIBCITE%%2%]). When the membrane potential reaches a threshold value
circuit, charged by (see Figure taken from [BIBCITE%%2%]). When the membrane potential reaches a threshold value 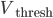 , a spike is emitted and the membrane potential is reset. In its basic form, the equation of the integrate and fire model is:
, a spike is emitted and the membrane potential is reset. In its basic form, the equation of the integrate and fire model is:
\begin{equation}
\tau_{\mathrm{m}} \frac{dV_{\mathrm{m}}(t)}{dt} = -V_{\mathrm{m}}(t) + RI(t)
\end{equation}
where is the resistance of the membrane, with .
To refine and be more precise, the neuronal input approximated as a fluctuating current but synaptic drives are better modelled by fluctuating conductances: the amplitudes of the post synaptic potentials (PSP) evoked by neurotransmitter release from pre-synaptic neuron depend on the post-synaptic depolarization level. A lot of study focuses now on this integrate-and-fire model with conductance-based synapses [Destexhe2001, Tiesinga2000, Cessac2008, Vogels2005]. The equation of the membrane potential dynamic is then:
\begin{equation}
\tau_{\mathrm{m}} \frac{dV_{\mathrm{m}}(t)}{dt} = (V_{\mathrm{rest}}-V_{\mathrm{m}}(t)) + g_{\mathrm{exc}}(t)(E_{\mathrm{exc}}-V_{\mathrm{m}}(t)) + g_{\mathrm{inh}}(t)(E_{\mathrm{inh}}-V_{\mathrm{m}}(t))
\end{equation}
When reaches the spiking threshold 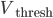 , a spike is generated and the membrane potential is held at the resting potential for a refractory period of duration . Synaptic connections are modelled as conductance changes: when a spike is emitted followed by exponential decay with time constants and for excitatory and inhibitory post-synaptic potentials, respectively. The shape of the PSP may not be exponential. Other shapes for the PSP can be used, such as alpha synapses , or double shaped exponentials synapses 2_{\mathrm{syn}}))(\mathrm{exp}(-t/\tau^1_{\mathrm{syn}}) - \mathrm{exp}(-t/\tau^2_{\mathrm{syn}}))" />. and are the reversal potentials for excitation and inhibition.
, a spike is generated and the membrane potential is held at the resting potential for a refractory period of duration . Synaptic connections are modelled as conductance changes: when a spike is emitted followed by exponential decay with time constants and for excitatory and inhibitory post-synaptic potentials, respectively. The shape of the PSP may not be exponential. Other shapes for the PSP can be used, such as alpha synapses , or double shaped exponentials synapses 2_{\mathrm{syn}}))(\mathrm{exp}(-t/\tau^1_{\mathrm{syn}}) - \mathrm{exp}(-t/\tau^2_{\mathrm{syn}}))" />. and are the reversal potentials for excitation and inhibition.
The synapse is a key element where the axon of a pre-synaptic neuron connects with the dendritic arbour of a post-synaptic neuron . It transmit the electrical influx emitted by neuron to . Synapses are crucial in shaping a network's structure, and their ability to modify their efficacy according to the activity of the pre and the post-synaptic neuron is at the origin of synaptic plasticity and memory retention in neuronal networks.
Synapses can be either chemical or electrical, but again, for a more exhaustive description,the latter here will be discarded. To focus only on the chemical synapses, the pre-synaptic neuron releases a neurotransmitter into the synaptic cleft which then binds to receptors located on the surface of the post-synaptic neuron , embedded in the plasma membrane. These neurotransmitters are stored in vesicles, regenerated continuously, but a too strong stimulation of the synapse may lead to a temporary lack of neurotransmitter, or to a saturation of the post-synaptic receptors on . This short-term plasticity phenomenon is called synaptic adaptation.
The type of neurotransmitter which is received to the post-synaptic neuron influences its activity. The synaptic current is cancelled for a given inversion potential: if this inversion potential is below 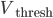 (the voltage threshold for triggering an action potential), the net synaptic effect inhibits the neuron, and if it is below, it excits the cell. Classical neurotransmitter such as glutamate leads to a depolarization (i.e. an increase of the membrane potential), and the synapse is said to be excitatory. In contrast, gamma-aminobutyric acid (GABA) leads to an hyper-polarization (a decrease of the membrane potential), and the synapse is said to be inhibitory. In general, a given neuron produces only one type of neurotransmitter, being either only excitatory or only inhibitory. This principle is known as the Dale's principle, and is a common assumption made in the models of neuronal networks.
(the voltage threshold for triggering an action potential), the net synaptic effect inhibits the neuron, and if it is below, it excits the cell. Classical neurotransmitter such as glutamate leads to a depolarization (i.e. an increase of the membrane potential), and the synapse is said to be excitatory. In contrast, gamma-aminobutyric acid (GABA) leads to an hyper-polarization (a decrease of the membrane potential), and the synapse is said to be inhibitory. In general, a given neuron produces only one type of neurotransmitter, being either only excitatory or only inhibitory. This principle is known as the Dale's principle, and is a common assumption made in the models of neuronal networks.
[captionpix imgsrc="http://www.yger.net/wp-content/uploads/2013/02/synapse.jpg" widht="500" captiontext="Top: schematic illustration of a synaptic contact between two neurons. The axon of pre-synaptic neuron establishes a synapse with a dendrite of post-synaptic neuron $B$. Bottom: detail of the synaptic cleft. Neurotransmitters stored in vesicles are liberated when the pre-synaptic membrane is depolarized, and then docked onto receptors of "]
[Bibtex]
@ARTICLE{Feltham2008,
author = {Feltham, D.L.},
title = {Sea Ice Rheology},
year = {2008},
abstract = {The polar oceans of Earth are covered by sea ice. On timescales much
greater than a day, the motion and deformation of the sea ice cover
(i.e., its dynamics) are primarily determined by atmospheric and
oceanic tractions on its upper and lower surfaces and by internal
ice forces that arise within the ice cover owing to its deformation.
This review discusses the relationship between the internal ice forces
and the deformation of the ice cover, focusing on representations
suitable for inclusion within global climate models. I first draw
attention to theories that treat the sea ice cover as an isotropic
continuum and then to the recent development of anisotropic models
that deal with the presence of oriented weaknesses in the ice cover,
known as leads.},
doi = {10.1146/annurev.fluid.40.111406.102151},
file = {Feltham2008.pdf:Feltham2008.pdf:PDF},
publisher = {Annual Reviews}
}[Bibtex]
